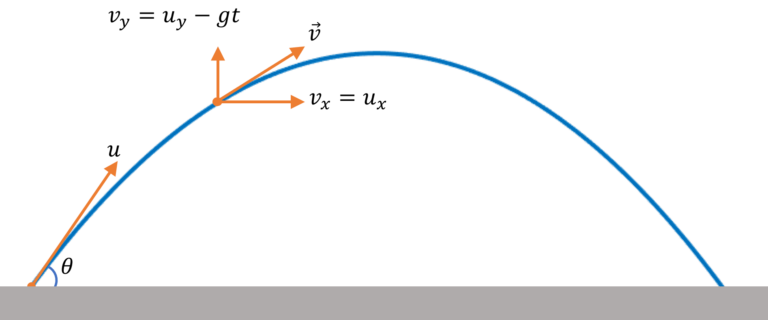
Recall, the equations of motion of the projectile for horizontal and vertical direction

Horizontal Direction : $x = u_x t$ $=u \cos \theta t$

Vertical Direction : $y = u_y t -$ $\cfrac{1}{2} gt^2$ $=u \sin \theta -$ $\cfrac{1}{2} gt^2$

Since we want the equation of trajectory $y = f(x)$, let’s go ahead and eliminate time variable $t$ from the two equations which gives us $y = \tan \theta x – \cfrac{g}{2u^2 \cos^2 \theta} x^2$

Now, let’s say that you have been given the equation of trajectory of the projectile as $y =$ $ax -$ $bx^2$, can you determine angle of projection, maximum height and range

Angle of Projection = $\left.\cfrac{dy}{dx}\right|_{t=0}$

Maximum Height: Find $x_{top}$ for which $\cfrac{dy}{dx} =0$, then maximum height, $H=$ $ax_{top}$ $- bx_{top}^2$

Range: Find $x$ for which $y=0$ (other than $x=0$) and that will be the range

With that, now let’s find out the radius of curvature at different points along the trajectory.