For NSEP (IOQP) 2022-2023 part A-1, click here
Note that there are 60 questions in total (48 in A1 and 12 in A2), with each question in A1 carrying 3 marks and each question in A2 carrying 6 marks, for a total of 216 marks. Total allotted time is 120 mins (2 hrs)
A-2
ANY NUMBER OF OPTIONS 4, 3, 2, OR 1 MAY BE CORRECT
MARKS WILL BE AWARDED ONLY IF ALL THE CORRECT OPTIONS ARE BUBBLED
49) In an isolated asteroid of radius $R$ and uniform density $\rho$, a spherical cavity of diameter $AC=R$ is excavated, where $C$ is center of asteroid. Choose correct alternative(s)
(a) A ball just dropped from $A$ will strike $C$ with speed $v = 2 R \sqrt{\cfrac{\pi \rho G}{3}}$
(b) A ball dropped from $A$ will reach $C$ after time $t = \sqrt{\cfrac{3}{\pi \rho G}}$
(c) Acceleration of ball dropped from $A$ varies as its distance from $O$ (center of cavity)
(d) Weight of a body placed at $B$ (diametrically opposite to $A$) on surface of asteroid decreased by a factor $\cfrac{7}{8}$ due to excavation of cavity.
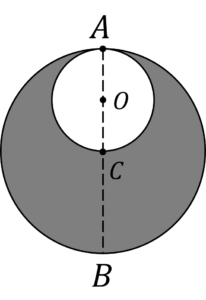
Correct answers are A) & B)
A) Potential at $A$, $V_A = \cfrac{3}{2} \cfrac{G \cfrac{4}{3} \pi R^3}{R} – \cfrac{G \cfrac{4}{3} \pi (R/2)^3}{R/2}$ $=\cfrac{5}{3} \pi \rho G R^2$
Potential at $C$, $V_C = \cfrac{G \cfrac{4}{3} \pi R^3}{R} – \cfrac{G \cfrac{4}{3} \pi (R/2)^3}{R/2}$ $=\pi \rho G R^2$
i.e. Gravitational potential difference between $A$ and $B$ $=\cfrac{2}{3} \pi \rho G R^2$
Now since the mechanical energy would remain conserved as the particle moves from $A$ to $B$
$\Delta U + \Delta K = 0$
or $v = 2 R \sqrt{\cfrac{\pi \rho G}{3}}$
B) To establish time taken by the particle to go from $A$ to $C$, let’s first find the acceleration of the particle when it is at a distance $x$ from $A$
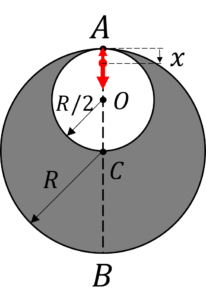
$F_{net,x} = ma_x$ $= \cfrac{G \rho \cfrac{4}{3} \pi (R-x)^3}{(R-x)^2} – \cfrac{G \rho \cfrac{4}{3} \pi (R/2-x)^3}{(R/2-x)^2}$
$a_x= \cfrac{2}{3} G \rho \pi R$
Now, $x = u_x t + \cfrac{1}{2} a_x t^2$
$u_x = 0$
$\implies t = \sqrt{\cfrac{3}{\pi \rho G}}$
C) As seen above $a_x$ is constant, So C) is NOT correct
D) $F_B = \cfrac{17}{18} \cfrac{4}{3} m \pi G R$, hence D) is NOT correct
50) A small positively charged ball of mass $m$ is suspended by a long insulating thread of negligible mass. Other positively charged small ball is moved very slowly from a large distance (along horizontal direction) until it is at original position $A$ of first ball. As a result the first ball rises by $h$ to position $B$ such that $h << l$. Choose the correct statement(s)
(a) Electrostatic energy of system of changes is $2 \ mgh$.
(b) Total work done on system to bring two balls in their final position is $mgh$.
(c) Total work done on the system to bring two balls in their final position is $3 \ mgh$
(d) Work done on system to bring two balls in their final position does not depend on the magnitude of charges explicitly.
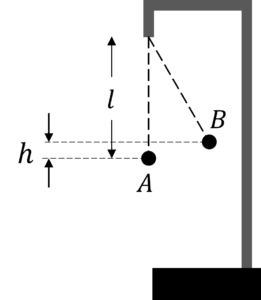
Correct answers are A), C) and D)
51) A rope of mass $m$ and length $L$ is suspended vertically. A mass $M$ is suspended from bottom of the rope. A transverse wave is produced on the rope, which travels the length of rope in time $t$. Choose the correct statement(s)
(a) $t = 2 \sqrt{\cfrac{L}{mg}} (\sqrt{M+m} – \sqrt{M})$
(b) For $m << M$, the time $t = \sqrt{\cfrac{mL}{Mg}}$
(c) For $M=0$ (i.e. no mass hanging) the time $t = \sqrt{\cfrac{L}{g}}$
(d) Time to travel the lower half of the rope by the wave is less than that to travel the upper half.
Correct answers are A), B),
52) A long solenoid having $1000$ turns per meter carries a current of $1A$. It has a soft iron core of $\mu_r = 1000$. The core is heated beyond the Curie temperature ($T_c$). Then
(a) The $H$ field in the solenoid is nearly unchanged but the $B$ field decreases drastically.
(b) The $H$ and $B$ fields in the solenoid are nearly unchanged
(c) The magnetization in the core reverses direction.
(d) The magnetization in the core diminishes by a factor of about $10^8$
Correct answer is A)
53) A hydrogen atom is in ground state ($n=1$). The magnetic field produced by revolving electron, at centre of atoms is $B_o$. Atom is excited to state $n=4$. According to Bohr model, the correct alternative(s) is/are
(a) Magnetic field at centre of atom for ($n=4$) becomes $B_4 = \cfrac{B_o}{64}$
(b)Energy absorbed by atom in going from ($n=1$) to ($n=4$) is $12.75 \ eV$
(c) Change in magnitude of angular momentum of electron is $\cfrac{3h}{2 \pi}$
(d) Assume that this excited atom ($n=4$) is at rest and it makes transition to ground state ($n=1$) in a single quantum jump of an electron. (Take mass of atom $M_H = 1.67 \times 10^{-27}$ \ Kg), the recoil speed of atom will be nearly $v = 4.1 \ ms^{-1}$
Correct answers are B), C), & D)
54) In an experimental setup to study the photoelectric effect a point source of light of power $3.2 \ mW$ is used. The source emits monoenergetic photons of energy $5 \ eV$ and is located at a distance $d = 0.8 \ m$ from center of a stationary metallic sphere of work function $W = 3.0 \ eV$. The radius of the sphere is $R = 8 \ mm$. Assume that the sphere is isolated and photoelectrons are instantly swept away after emission. Also, assume that the efficiency of photoelectric emission is one for every $10^6$ photons. In the present setup
(a) The de Broglie wavelength of the fastest moving photoelectron is nearly $8.7 \ A^{\circ}$
(b) It is observed that after some time emission of photoelectrons from the surface of the metal sphere is stopped, the charge on sphere just when the electron emission stops is $64 \pi \epsilon_0 \times 10^{-3} \ C$
(c) Time after which photo electric emission stops is nearly $111 \ s$
(d) The light source emits $4 \times 10^{15}$ photons per sec
Correct answers are A), B), C), D)
55) Two identical Carnot (cycles) engines operate between maximum and minimum temperatures $T_1$ and $T_2$ and volume limits, $V_a$, $Vba$, $V_c$ & $V_d$ as shown in the figure. Given that $\cfrac{V_c}{V_a} = e^3$ and $\cfrac{T_1}{T_2} = e$ ($e$ is the base of natural logarithm). Engine $1$ operates on monoatomic gas while the engine $2$ on diatomic gas. Choose correct alternatives
(a) Ratio of volumes $\cfrac{V_{b,1}}{V_{b,2}} = e$
(b) Ratio of work done per cycle for the two is $\cfrac{W_1}{W_2} = 3$
(c) Ratio of work per cycle for the two is $\cfrac{W_1}{W_2} = 1$
(d) Ratio of efficiencies ($\eta$) of two engines $\cfrac{\eta_1}{\eta_2} = 1$

Correct answers are A), B) & D)
56) In a certain machine two steel plates are separated by a hardened steel cylindrical roller (see fig). In operation, the plates move back and forth horizontally, perpendicular to the axis of roller, and the roller rolls freely between plates without slipping on either one. At a particular instant plate $A$ is moving with speed of $18 \ cm \ sec^{-1}$ to the right and an acceleration of $30 \ cm \ sec^{-2}$ to the left, and the plate $B$ is moving with a speed of $6 \ cm \ sec^{-1}$ to the right and an acceleration of $8 \ cm \ sec^{-2}$ to the left. At that instant, for the roller
(a) Its angular speed is $3 \ rad \ sec^{-1}$ clockwise
(b) Its angular acceleration is $6 \ rad \ sec^{-2}$ clockwise
(c) The linear speed of its axis is $12 \ cm \ sec^{-1}$ towards right
(d) The linear acceleration of its axis is $20 \ cm \ sec^{-2}$ towards left
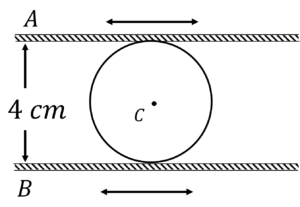
Correct answers are A) & C)

Recall that velocity of any point, $P$, on a rigid body under complex motion (translational + rotational) motion can be written as
$\overrightarrow{v}_p = \overrightarrow{v}_{cm} + \overrightarrow{\omega} \times \overrightarrow{r}_p$
So $v_A = v_C + \omega R$
$v_B = v_C – \omega R$
Solving we get $v_C = 12 \ cm \ sec^{-1}$ & $\omega = 3 \ rad \ sec^{-1}$
Similarly $a_A = a_{cm} + \alpha R$
$a_B = a_{cm} – \alpha R$
Solving we get
$a_C = 19 \ cm \ sec^{-2}$
& $\omega = 5.5 \ rad \ sec^{-2}$
57) Each of 9 sides of frame ACDEFB has resistance $R$ (Nine in all). A current I enters at $A$ and leaves at $B$. Choose the correct alternatives
(a) Currents in branches CD and EF are zero
(b) Currents in branches CE and DF are each equal to $\cfrac{4}{11} I$
(c) Effective resistance between $A$ and $B$ is $\cfrac{15}{11}R$
(d) Effective resistance between $A$ and $B$ is $\cfrac{3}{4} R$
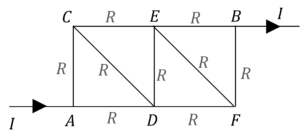

Using Delta-Y transformation we can reduce the given configuration to the following
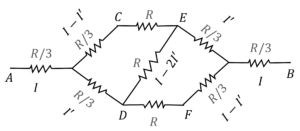
Now writing kirchoff’s law for the loop formed by CED, we will get $I’ = \cfrac{4}{11} I$
Now writing potential difference between $A$ and $B$ we get $\Delta V_{A \rightarrow B} = \cfrac{15}{11} IR$
and equating it to $I R_{eq}$, we get
$R_{eq} = \cfrac{15}{11} R$
58) A long uniform rod of length $L$ and mass $M$ is pivoted vertically on a horizontal, frictionless pivot at its lower end. The rod is released from rest in its vertical position $OA$ (see figure). It falls off without slipping at $O$. At the instant the rod is horizontal,
(a) Its angular speed is $\sqrt{\cfrac{3g}{L}}$
(b) Magnitude of its angular acceleration is $\cfrac{3g}{2L}$
(c) Acceleration of its center of mass $\overrightarrow{a}_{CM} = – \cfrac{3g}{4} \hat{j}$ ($\hat{j}$ unit vector in $Y$ direction)
(d) Reaction force at pivot $= \cfrac{Mg}{4} \hat{j}$ (Take X, Y axis as shown)

Correct answers are A), B)
59) There are four layers of glass plates, placed on top of each other such that bottom one has thickness $a_1$ and refractive index $n_1 = 2.7$. Next one has thickness $a_2$ and refractive index $n_2 = 2.43$. The third one and the top one have thickness $a_3$ and $a_4$ and refractive indices $n_3$ and $n_4$ respectively. Three rays starting at the same moment from $A_1$, $A_2$ and $A_3$ reach points $B_2$, $B_3$, $B_4$ at the same time, with their angles of incidence being critical angles. You are given $A_1 B_1$ $= A_2 B_2$ $= A_3 B_3$ $= A_4 B_4$ $=b = 10 \ mm$. Choose the correct statement(s).
(a) $n_3 = 1.968$
(b) $n_4 = 1.291$
(c) $a_2 = 7.243 \ mm$
(d) $a_3 = 11.51 \ mm$
[In four significant figures]
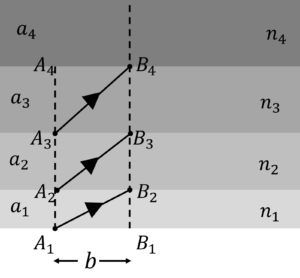
Correct answers are A), B) & D)
Time taken by rays (to go from starting point $A$ to $B$ respectively) in all 3 mediums is same, meaning
$\cfrac{A_1 B_2}{v_1} = \cfrac{A_2 B_3}{v_2} = \cfrac{A_3 B_4}{v_3}$
Now,
$A_1 B_2 = \sqrt{a_1^2 + b^2}$
$A_2 B_3 = \sqrt{a_2^2 + b^2}$
$A_3 B_4 = \sqrt{a_3^2 + b^2}$
& $v_1 = \cfrac{c}{n_1}$
$v_2 = \cfrac{c}{n_2}$
$v_3 = \cfrac{c}{n_3}$
$\implies$ $n_1 \sqrt{a_1^2 + b^2} = n_2 \sqrt{a_2^2 + b^2} = n_3 \sqrt{a_3^2 + b^2}$ $\cdots$ (1)
Additionally, it is given that all 3 rays are incident on the medium interfaces at critical angles. meaning
$n_1 \cfrac{b}{\sqrt{a_1^2 + b^2}} = n_2$ $\cdots$ (2)
$n_2 \cfrac{b}{\sqrt{a_2^2 + b^2}} = n_3$ $\cdots$ (3)
$n_3 \cfrac{b}{\sqrt{a_3^2 + b^2}} = n_4$ $\cdots$ (4)
From equation (2) we can write
$\sqrt{a_1^2 + b^2} = \cfrac{n_1}{n_2} b $
And so from equation (1), we will get
$\sqrt{a_2^2 + b^2} = \cfrac{n_1^2}{n_2^2} b$
or $a_2 = 7.240 \ mm$
and from equation (3) we get
$n_3 = \cfrac{n_2^3}{n_1^2}$ $= 1.968$
Similarly we can solve for $a_3$ and $n_4$
60) A thin and infinitely long metal sheet of appreciable finite width $b$ carrying current $I$ (distributed uniformly through out of its cross section) parallel to its length is placed in an external magnetic field $B_e$, parallel to its plane and perpendicular to the direction of current
(a) The thin metal sheet experiences a mechanical pressure $P = \cfrac{I B_e}{b}$ perpendicular to its face.
(b) The direction of the pressure does not change if the direction of current is reversed.
(c) In case the external magnetic field $B$, is switched off, a magnetic field $B = \cfrac{\mu_0 I}{2b}$ is observed parallel to the plane of the sheet but perpendicular to the direction of current.
(d) The magnetif field produced in part (c) is $B = \cfrac{2 \mu_0 I}{2b}$
Correct answers are A), C)
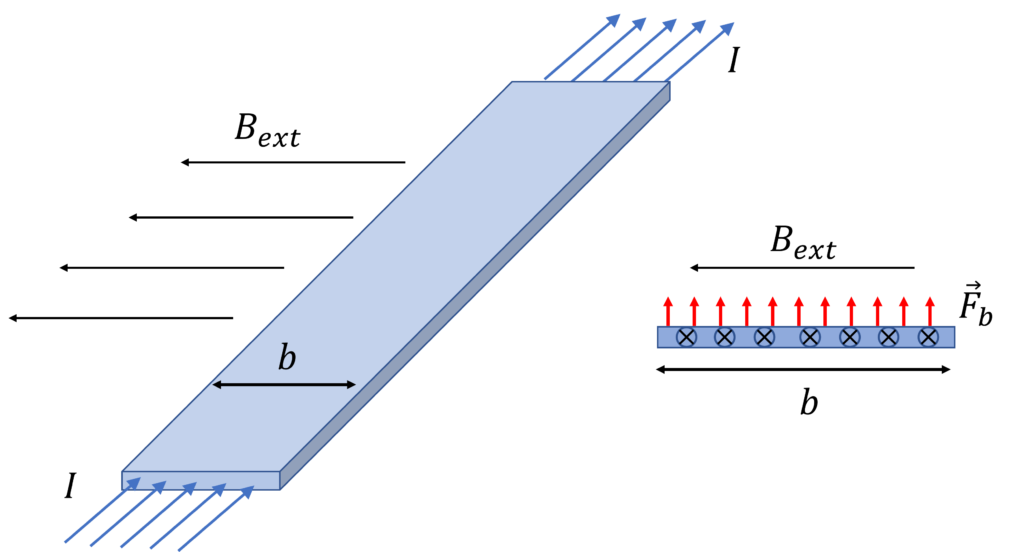
Force on a segment of sheet of length $L$ and width $b$, $F_b$ will be
$F_b = I L B_{e}$
so, pressure or force per unit area on this section of the sheet will be $\cfrac{F_b}{bL}$ $= \cfrac{I B_e}{b}$
As for the direction of force (& resulting pressure), as we reverse direction of the current, direction of force (& hence pressure) will get reversed
Now, if we were to switch off the external magnetic field, $B_e$, then using the given information that $b$ is significant, choose an amperian loop DEFG as shown
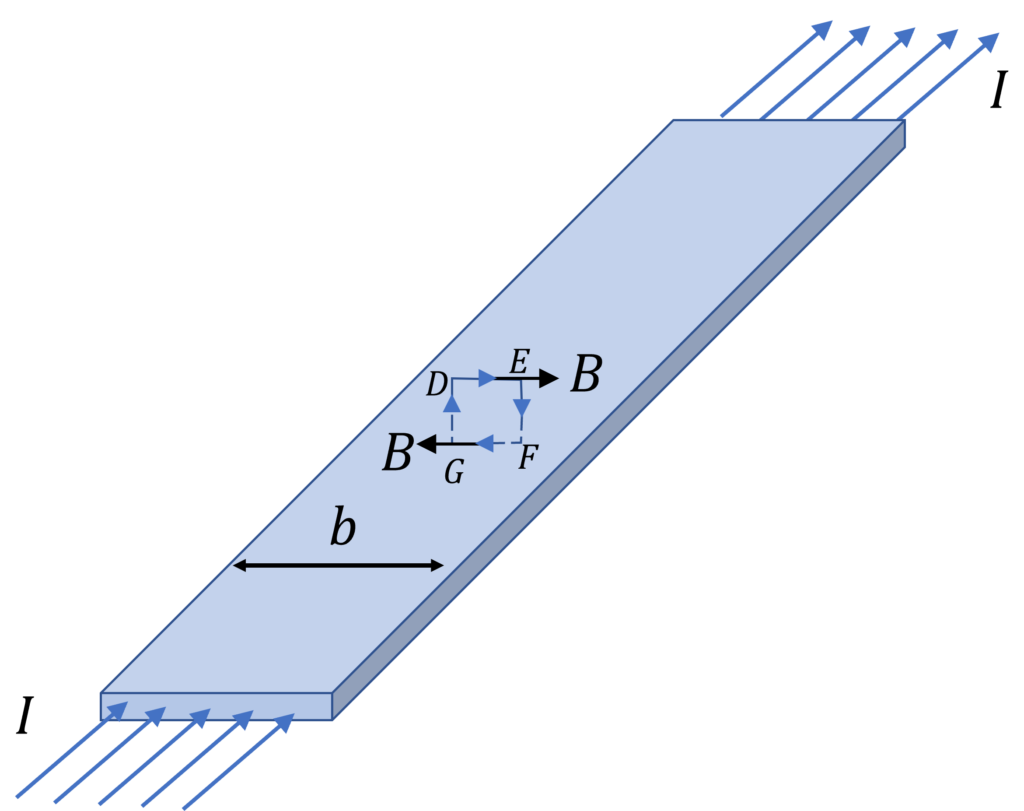
and we can argue using symmetry and right hand thumb rule that the magnetic field will be to the right, above the plate and to the left, below the plate
then using the ampere’s law
$\oint \overrightarrow{B}.\overrightarrow{dl} = \mu_0 i_{enc}$
we get
$B = \cfrac{\mu_0 I}{2b}$




