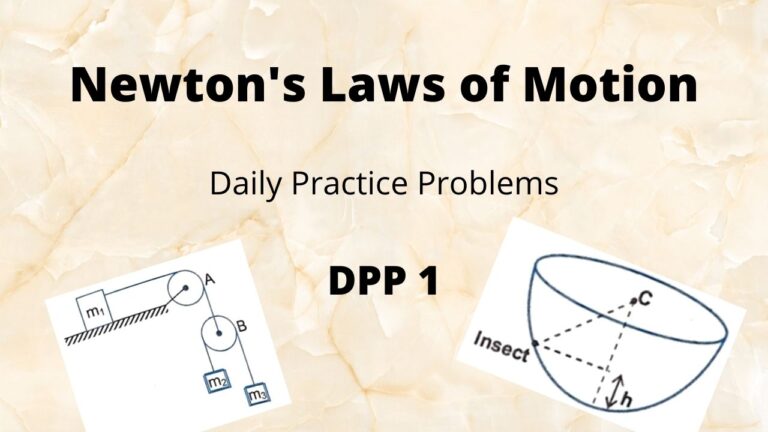
Q1 A uniform heavy rod of weight $W$, cross sectional area $A$ and length $L$ is hanging from a fixed support. Young’s modules of the material of the rod is $Y$; find the elongation of the rod due to its own weight and potential energy stored, Neglect the lateral contraction.
$\cfrac{WL}{2 A Y}$, potential energy $ = \cfrac{1}{6} \cfrac{W^2 l}{AY}$
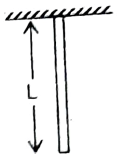
Q2 Two light wires made up of same material (Young Modulus, $Y$) have length $L$ each and radii $R$ and $2R$ respectively; they are joined together and suspended from a rigid support. Now a weight $W$ attached to the free end of joint wire as shown in figure. Find the elastic potential energy stored in the system due to extension of wire.
$\cfrac{5W^2 L}{8 \pi R^2 Y}$
Q3 A long uniform rope of mass $M$ is suspended from a rigid end, calculate longitudinal strain due to its own wt. Diameter and young’s modulus of rope are $D$ and $Y$ respectively.
$\cfrac{2Mg}{\pi D^2 Y}$