Types of Projectile Motion

Let’s begin with projectile motion definition, followed by a review of different types of projectile motion after which we will examine the equations of motion of a projectile

Projectile Motion Definition

Projectile motion is the motion of an object thrown up in the air at an angle from the horizontal (or ground) with object moving under gravitational acceleration pointed vertically downwards, Now depending on the angle of projection, ‘$\theta$’ we can classify the projectile motion as oblique projectile motion also referred to as angular projectile motion for $0 < \theta < 90^\circ$ or vertical projectile motion ($\theta = 90^\circ$). 

Now, if the projectile is thrown at angle $\theta = 0^\circ$, it is referred to as horizontal projectile motion

So, before we get into the equations of motion for the above three scenarios, let’s visualize the projectile motion.

Oblique Projectile Motion

Note that 

  • while the initial velocity is same for all 4 projectiles, time of flight is NOT same for all four
  • for projection angles, $\theta = 30^\circ$ and $\theta = 60^\circ$, range (horizontal distance covered by projectile) is same
  • range is maximum for projection angle $\theta = 45^\circ$
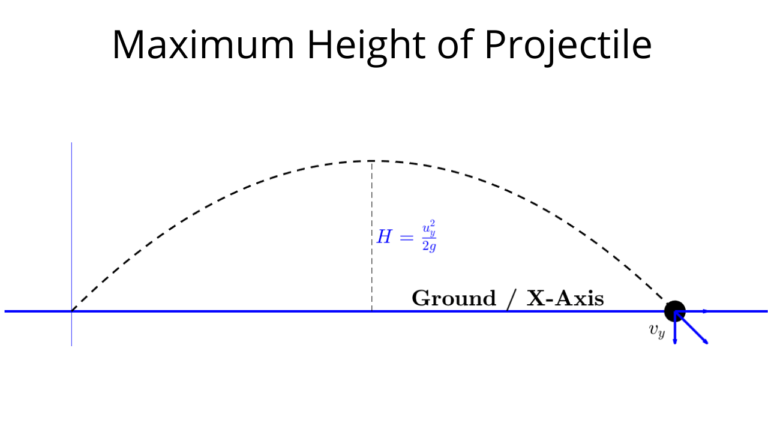
  • for same initial velocity, maximum height achieved by the projectile increases with projection angle and is maximum for vertical projection
  • horizontal component of the velocity does NOT change as there is no acceleration in the horizontal direction.

Now let’s quickly visualize the horizontal projectile motion, before we get into equations of motion for a projectile

Horizontal Projectile Motion

Note that the initial speed in the vertical direction is zero.

With this visualization, now let’s get into the equations of motion for a projectile

Equations of Motion (Eq) of Projectile

We will begin with equations of motion, eq, of projectile for oblique projectile motion and we will then see how these equations change for $\theta = 0^\circ$ (horizontal projectile motion) and $\theta = 90^\circ$ (Vertical Projectile Motion)

So, to begin with, note that, there is no acceleration in the horizontal direction (if we ignore air drag) but there is acceleration due to gravity in the vertical direction, with ‘$g$’ pointed downwards.

Now, we can use the equations of motion for one dimension, i.e., $v =$ $u +$ $at$ and $\Delta s = ut + \cfrac{1}{2} at^2$ for motion in the horizontal direction and also for motion of the projectile in vertical direction.

In horizontal direction, only meaningful equation out of the above two equations is:

$\Delta x$ or $x=u_xt$, 

where $x$ is the displacement in the horizontal direction assuming origin is at the point of launch of the projectile and $u_x$ is the speed of the projectile in the horizontal direction, which will not change with time as $a_x = 0$ or there is no acceleration in the horizontal direction 

In vertical direction, we can write the two kinematic equations as

$v_y = u_y + a_y t$ or $v_y = u_y – gt$

where u_y is the initial speed in the vertical direction or we can say, vertical component of initial velocity $\overrightarrow{u}$

$\Delta y$ or $y = u_yt + \cfrac{1}{2} a_y t^2$ or $y = u_yt – \cfrac{1}{2} g t^2$

$u_x = u \cos \theta$ and $u_y = u \sin \theta$

Note that, for projection angle $\theta = 90^\circ$, $u_x = 0$, meaning $x = 0$ (vertical projectile motion) and for $\theta = 0^\circ$, $u_y = 0$.

With that, now let’s explore the range, maximum height, trajectory, radius of curvature and other features of projectile motion

Projectile Motion | Important Questions | JEE PYQs

    Kinematics Overview – What you need to know for JEE Main and JEE Advanced